box cox distribution The Box-Cox transformation is a particulary useful family of transformations to convert a non-normal behaving data set into an approximately a normal distribution. $70.00
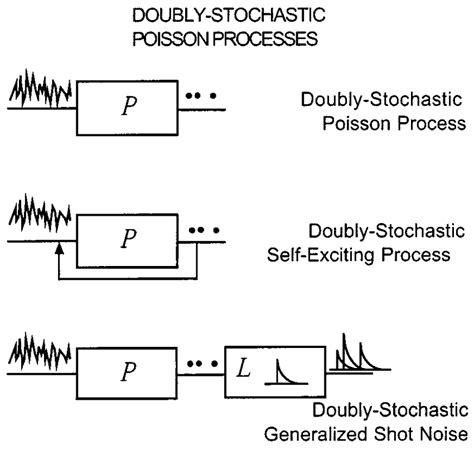
0 · doubly stochastic poisson process
1 · cox regression equation
2 · box cox transformation negative values
3 · box cox transformation lambda values
4 · box cox t distribution
5 · box cox plot interpretation
6 · box cox normal distribution
7 · box cox lambda meaning
$57.94
In statistics, the Box–Cox distribution (also known as the power-normal distribution) is the distribution of a random variable X for which the Box–Cox transformation on X follows a truncated normal distribution. It is a continuous probability distribution having probability density function (pdf) given by for y > 0, where m is the location parameter of the distribution, s is the dispersion, ƒ is the family . The Box-Cox transformation is a particulary useful family of transformations to convert a non-normal behaving data set into an approximately a normal distribution. Box-Cox transformation is a statistical technique that transforms your target variable so that it resembles a normal distribution. Here’s how to implement it in Python. . The Box-Cox transformation is a statistical technique for transforming non-normal data into a normal distribution. This transformation can improve the accuracy of predictions made using linear regression.
The main objective in the analysis of Box-Cox transformation model is to make inference on the transformation parameter λ, and Box and Cox(1964) considered two approaches.What is the Box Cox Transformation? A Box Cox Transformation is a simple calculation that may help your data set follow a normal distribution. Box Cox transformation was first developed by . In the literature, Box–Cox transformations are applied to basic distributions, e.g., the cubic root transformation of chi-squared variates is used for acceleration to normality (cf. .
Examples of the Box-Cox transformation versus log (x) for −2 < λ < 3. The bottom curve corresponds to λ = −2 and the upper to λ = 3. Another important use of variable transformation is to eliminate skewness and other distributional . The Box-Cox transformation is a family of power transformations, invented by George Box and Sir David Roxbee Cox in 1964, designed to stabilize variance and make the .In statistics, the Box–Cox distribution (also known as the power-normal distribution) is the distribution of a random variable X for which the Box–Cox transformation on X follows a truncated normal distribution.
doubly stochastic poisson process
A Box Cox transformation is a transformation of non-normal dependent variables into a normal shape. Normality is an important assumption for many statistical techniques; if your data isn’t normal, applying a Box-Cox means that you are able to run a broader number of tests. The Box-Cox transformation is a particulary useful family of transformations to convert a non-normal behaving data set into an approximately a normal distribution. Box-Cox transformation is a statistical technique that transforms your target variable so that it resembles a normal distribution. Here’s how to implement it in Python. Written by Andrew Plummer
The Box-Cox transformation is a statistical technique for transforming non-normal data into a normal distribution. This transformation can improve the accuracy of predictions made using linear regression.The main objective in the analysis of Box-Cox transformation model is to make inference on the transformation parameter λ, and Box and Cox(1964) considered two approaches.
large metal project enclosure
What is the Box Cox Transformation? A Box Cox Transformation is a simple calculation that may help your data set follow a normal distribution. Box Cox transformation was first developed by two British statisticians, namely George Box and Sir David Cox. In the literature, Box–Cox transformations are applied to basic distributions, e.g., the cubic root transformation of chi-squared variates is used for acceleration to normality (cf. also Normal distribution), and the square-root transformation stabilizes variances of Poisson distributions (cf. also Poisson distribution). These results are .Examples of the Box-Cox transformation versus log (x) for −2 < λ < 3. The bottom curve corresponds to λ = −2 and the upper to λ = 3. Another important use of variable transformation is to eliminate skewness and other distributional features that complicate analysis. Often the goal is to find a simple transformation that leads to normality. The Box-Cox transformation is a family of power transformations, invented by George Box and Sir David Roxbee Cox in 1964, designed to stabilize variance and make the data more closely conform to a normal distribution.
In statistics, the Box–Cox distribution (also known as the power-normal distribution) is the distribution of a random variable X for which the Box–Cox transformation on X follows a truncated normal distribution.A Box Cox transformation is a transformation of non-normal dependent variables into a normal shape. Normality is an important assumption for many statistical techniques; if your data isn’t normal, applying a Box-Cox means that you are able to run a broader number of tests. The Box-Cox transformation is a particulary useful family of transformations to convert a non-normal behaving data set into an approximately a normal distribution.
Box-Cox transformation is a statistical technique that transforms your target variable so that it resembles a normal distribution. Here’s how to implement it in Python. Written by Andrew Plummer
The Box-Cox transformation is a statistical technique for transforming non-normal data into a normal distribution. This transformation can improve the accuracy of predictions made using linear regression.The main objective in the analysis of Box-Cox transformation model is to make inference on the transformation parameter λ, and Box and Cox(1964) considered two approaches.What is the Box Cox Transformation? A Box Cox Transformation is a simple calculation that may help your data set follow a normal distribution. Box Cox transformation was first developed by two British statisticians, namely George Box and Sir David Cox. In the literature, Box–Cox transformations are applied to basic distributions, e.g., the cubic root transformation of chi-squared variates is used for acceleration to normality (cf. also Normal distribution), and the square-root transformation stabilizes variances of Poisson distributions (cf. also Poisson distribution). These results are .
Examples of the Box-Cox transformation versus log (x) for −2 < λ < 3. The bottom curve corresponds to λ = −2 and the upper to λ = 3. Another important use of variable transformation is to eliminate skewness and other distributional features that complicate analysis. Often the goal is to find a simple transformation that leads to normality.
cox regression equation
large outdoor electrical box cover
large metal trunk box
Simple yet classical design can be yours with a gorgeous vintage-style shower enclosure set. These sleek, complete packages offer durable design and matching premier finishes on every component, combined with good looks and trouble-free performance.
box cox distribution|box cox plot interpretation